Part 1:
What is Load Factor?
Load factor is a numerical value that indicates the ratio of lift to weight. It tells you how much extra force your drone is working against to stay in flight compared to simply hovering.
Why Turns Add Stress
In straight, level flight, the load factor is 1. This means the drone is only generating enough lift to balance its weight, similar to cruising on a flat road without added stress.
When the drone turns, it banks or tilts to the side. This banking increases the demands on the drone because it requires more lift to counteract the additional forces at play. The load factor quantifies this extra demand.
As the bank angle becomes steeper during a turn, the load factor rises because the drone needs to generate significantly more lift to maintain altitude.
In essence, the sharper the turn, the harder the drone has to work to stay stable in the air.
Why Load Factor This Matters
- Stress on the Drone: Higher load factors increase the stress on the drone’s structure, motors, and components.
- Battery Drain: Increased load means the drone has to work harder to maintain lift, draining the battery faster.
- Flight Control: Pilots need to manage steep banks carefully to avoid overloading the drone or losing control.
Influences on Load Factor
Wind Gusts & Turbulent Air
When flying a drone, gusty winds and turbulence can disrupt smooth airflow around the propellers, causing fluctuations in lift and an increased load factor.
Gusts can cause the drone’s lift to fluctuate, making it feel like it’s being pushed up or down unpredictably.
Gusty winds and turbulence cause the load factor to spike, meaning the drone experiences more force on its wings than usual. This extra force can strain the drone’s structure and impact its performance.
The drone might wobble, lose altitude, or become harder to control, especially with strong or frequent gusts.
Steep Climbs & Turns
Steep climbs and sharp turns both increase the load factor on a drone, requiring more effort from its propellers. During a steep climb, the propellers must work harder to generate additional lift, making the drone feel heavier as it fights against gravity. In sharp turns, the drone banks, and the centripetal force adds strain to the propellers, further raising the load factor. This increased effort can impact the drone’s stability and smoothness, particularly during aggressive maneuvers.
Payloads: Maximum Load Factor
Every drone is designed with a maximum load factor it can safely handle.
This value represents the highest level of stress that the drone’s structure can withstand without being damaged. The maximum load factor varies depending on the drone’s design, weight, and intended use. It’s an essential limit that operators must not exceed to ensure the drone’s integrity and safety during flight.
Flying a small UA near its maximum allowable weight can lead to shorter endurance, decreased maneuverability, and reduced speed.
Part 2:
How to Calculate Load Factor
This equation determines whether your drone can handle a turn without surpassing its max weight capacity and stalling.
Understand the Load Factor Equation
You’ll need to perform a straightforward calculation using two key factors related to your drone’s weight and the angle of turn you’re wanting it to achieve.. This equation helps determine whether your drone can handle the turn without surpassing its maximum weight capacity.
The term “Load Factor” means the extra force (or G-force) pushing on the drone compared to its weight when it’s in level flight. This isn’t the drone’s weight itself but rather how much harder the drone has to work to stay in the air because of the outward push it requires to make a given turn. The sharper the angle of turn, the more force required to achieve the turn without stalling.
Every sUAS has a payload maximum—the upper limit of weight it can safely carry—including the drone’s own weight, any additional equipment, and the forces it experiences during maneuvers. You can find this information in the manufacturer’s guidelines. Before executing any turns or maneuvers, make sure the calculated load factor doesn’t push your drone past this maximum, as exceeding it can cause structural damage or loss of control.
Step 1: Find Your Bank Angle & Load Factor on Chart
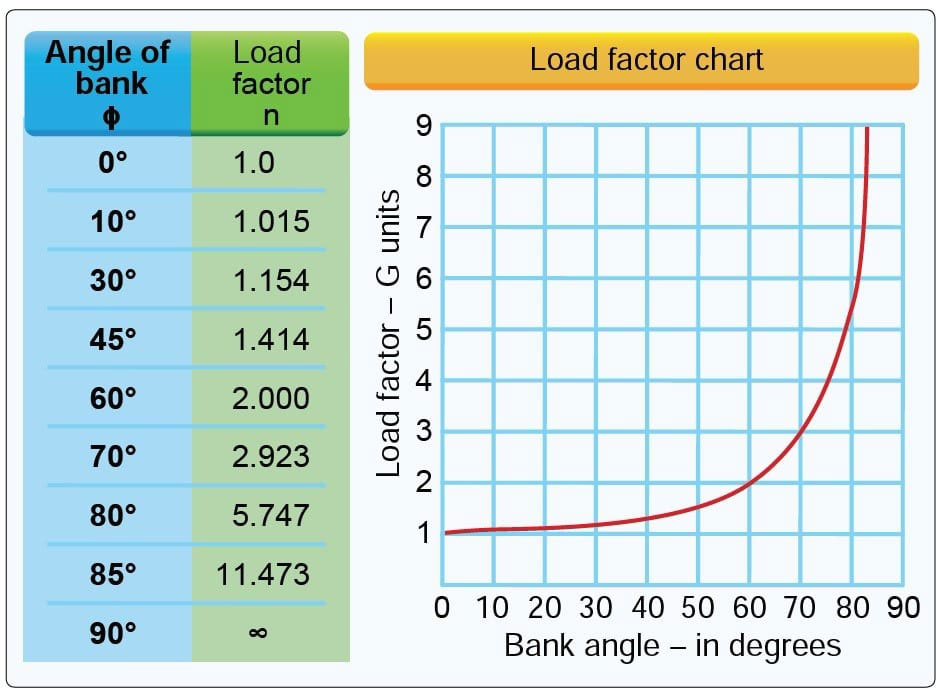
The below chart illustrates the relationship between bank angle and load factor for a drone. It shows how the load factor increases with steeper bank angles, impacting the drone’s effective load.
Chart Breakdown
- Blue/Green Table: This chart allows you to select a specific bank angle you wish to achieve (blue column) and the load factor associated with it (green column).
- Graph: The graph on the right is a visual chart showing exactly what the blue and green table on the left represents. You can see with the steep upward direction of the red line, as the angle increases (shown on the bottom X-Axis), the load factor increases (shown on the left Y-Axis)
Chart Numbers
- In straight and level flight, the load factor is 1.0 (just the drone’s weight).
- When a drone banks into a turn, centrifugal force increases, causing the drone to experience a load greater than its weight.
- The load factor increases as the bank angle gets steeper. For example:
- At a 0° bank (level flight), the load factor is 1.0.
- At a 30° bank, the load factor is 1.154 (meaning the drone feels 1.154 times its weight).
- At a 60° bank, the load factor jumps to 2.0 (the drone feels twice its weight).
Step 2: Calculate Load Factor
So now that we know how to find the key information on the chart, let’s do the equation.
Step 1: Select the Bank Angle from Column 1 (i.e 30°) and find the c
orresponding Load Factor in Column 2 (ie 1.154 corresponds to 30°)
Step 2: Multiply the weight of your sUAS by the load factor you just obtained from the chart.
or a 30° bank angle: Load factor = 1.145. Therefore, with a 3-pound drone, the effective load is 3 pounds x 1.145 = 3.435 pounds.
Part 3:
Load Factor Practice
3 Pound sUAS Load Factor at 30° 60° 80° Angles
Scenario:
Say you want to fly in a wooded area through trees, requiring turns at various degrees. Let’s find out how much of an angle we can go to without stalling.
Your sUAS weighs 3 pounds, and it has a max payload capacity of 10 pounds. What bank angles can your UA handle without stalling?
Remember, if your UA eventually gets so tilted when banking to achieve these angles, the propellors can no longer generate enough lift, and you stall. It’s at this point you’d need to regain control of your drone by reducing the angle of the turn.
The graphic below shows what those bank angles look like and the associated equation. Below the graphic we break down each equation at 30°, 60°, and 80°.
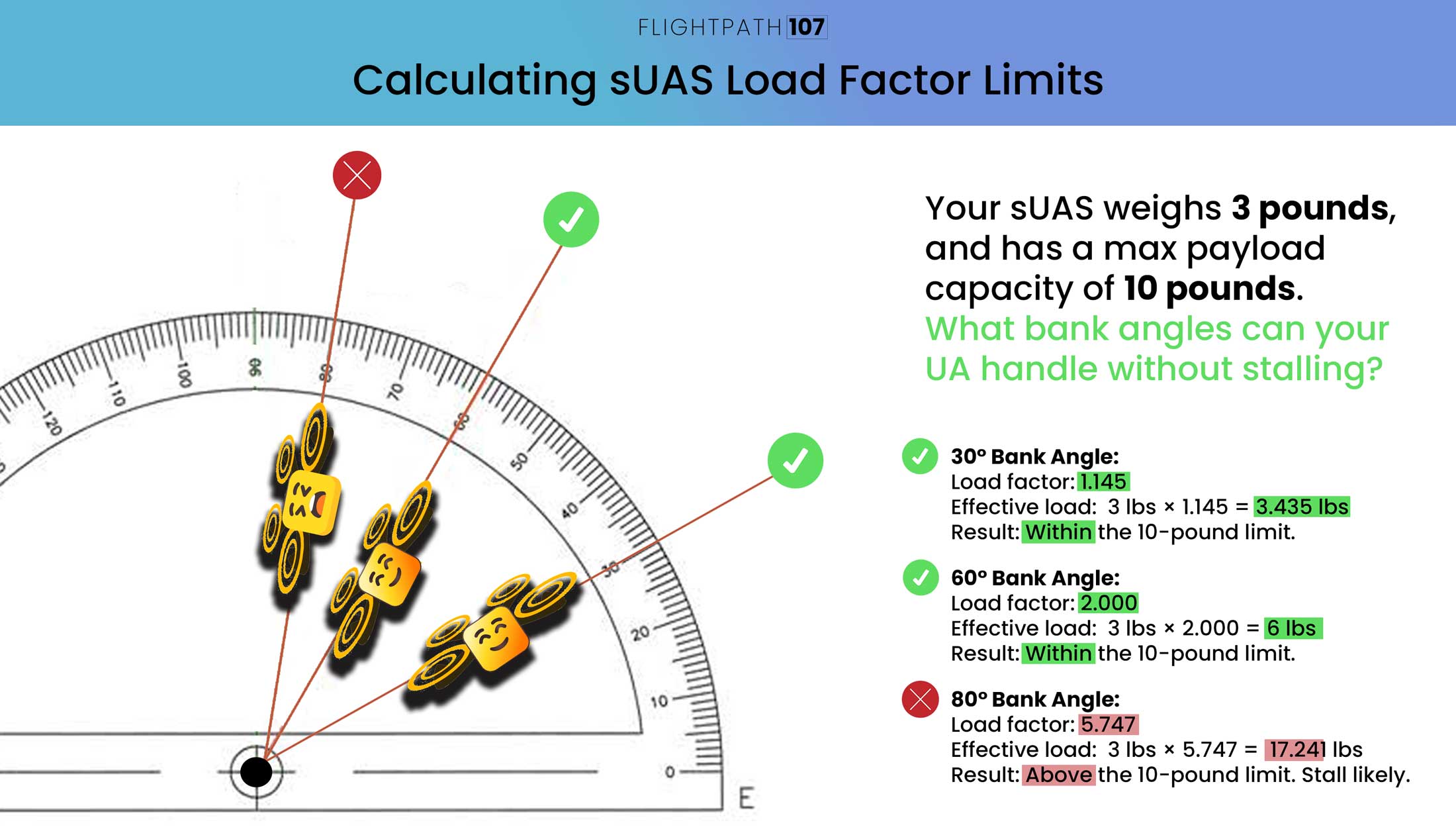
For a 30° bank angle:
Load factor = 1.145. Thus, with a 3-pound drone, the effective load is 3 pounds x 1.145 = 3.435 pounds, which is within the 10-pound limit.
For a 60° bank angle:
Load factor = 2. Thus, with a 3-pound drone, the effective load is 3 pounds x 2.0 = 6 pounds, which is within the 10-pound limit.
For a 80° bank angle:
Load factor = 5.747 . Thus, with a 3-pound drone, the effective load is 3 pounds x 5.747 = 17.241 pounds, which is well over the 10-pound limit. Your drone would be likely to stall.
Practice Quiz
Calculating UAS Load Requirements for Turns
To ensure your UAS can handle the forces during a turn, you’ll need to perform a simple calculation involving two key numbers: your UAS’s weight and the load factor from the below FAA chart. This number determines if the drone can handle the turn without exceeding its maximum weight capacity.
The chart, which includes different bank angles (e.g., 30°, 60°, and 80°), provides the load factors you need. On exam day, you’ll receive a printed version of this chart and a calculator. Here’s how to use these resources for your calculation:
FAA Load Factor Chart:
- X-Axis: Bank Angle (degrees) – Displays the angle at which the drone is banked during a turn.
- Y-Axis: Load Factor – Represents the multiple of the drone’s weight that the drone effectively experiences at different angles.
Be Aware: Load Factor Significantly Increases at 45°-50° Bank Angles
The load factor rises sharply around a 45°-50° bank angle.
At these steeper angles, the forces needed to keep the sUAS in the air increase quickly.
Essentially, the sUAS and its components start to feel much heavier. This is because the sUAS needs significantly more lift to maintain altitude while turning, and this need grows rapidly as the bank angle steepens.
Question You have Sample questions, above, then more questions here, then Practice questions, then the quiz. Too many question sections ! 🙂
When operating an unmanned airplane, the remote pilot should consider that the load factor on the wings may be increased any time:
A. the CG is shifted rearward to the aft CG limit.
B. the airplane is subjected to maneuvers other than straight-and-level flight.
C. the gross weight is reduced.
B. the airplane is subjected to maneuvers other than straight-and-level flight.
Explanation:
When an unmanned airplane (or any aircraft) is subjected to maneuvers other than straight-and-level flight, such as turns, climbs, or descents, the load factor on the wings increases. This is because these maneuvers require the wings to generate additional lift to counteract the increased gravitational forces acting on the aircraft. As the load factor increases, so does the stress on the wings and other structural components of the aircraft. Therefore, it’s important for remote pilots to consider the effects of load factor when maneuvering the aircraft to ensure safe operation.
Question
The importance and use of performance data to predict the effect on the aircraft’s performance of an sUAS. When operating an unmanned airplane, the remote pilot should consider that the load factor on the wings may be increased any time:
A. the CG is shifted rearward to the aft CG limit. [This wouldn’t increase load factor. If the airplane uses an elevator for pitch, this would actually DECREASE load factor.]
B. the airplane is subjected to maneuvers other than straight and level flight.
C. the gross weight is reduced. [Gross weight reduction would DECREASE load factor.]
Answer: B. the airplane is subjected to maneuvers other than straight and level flight.
Question
The importance and use of performance data to predict the effect on the aircraft’s performance of an sUAS. (Refer to FAA-CT-8080-2H, Figure 2.) If an unmanned airplane weighs 33 pounds, what approximate weight would the airplane structure be required to support during a 30° banked turn while maintaining altitude?
A.34 pounds.
B. 47 pounds.
C. 38 pounds.
Answer: C
[Explanation: In a turn of 30 degrees of bank and while maintaining level flight (no altitude loss because you slightly pitched up), you will have a 1.154 load factor. This means that in this turn you will be feeling like you are pulling 1.154 G’s. 33 pounds x 1.154 = 38.082 pounds.
Practice Quiz
quiz title is Payload Shifts, but that’s not part of this section.
question 4, it’s 57.57 pounds, so wouldn’t it need to support 58? 57 wouldn’t be enough?
question 6 the correct answer isn’t one of the options. It’s 46.41 pounds




